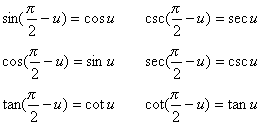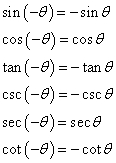5.5 -Multiple-Angle and Product-Sum Formulas

-This Blog will be written in Violet-

Double-Angle Formulas : The most commonly used multiple-angle formulas. They are used often, so you should learn them.

sin2u = 2sinu cosu

cos 2u = cos^2u - sin^2u

=2cos^2u - 1

=1 - 2sin^2u

tan2u = (2tanu) / ( - tan^2u)

A visual proof for the Double-Angle Formula for Sine:

Power-Reducing Formulas: Can be obtained through the double-angle formulas

Sin^2X = (1-cos2X) / (2)

Cos^2X = (1 + cos2X) / (2)

Tan^2X = (1 - cos2X) / (1 + cos 2X)

Half-Angle Formulas : Can be derived from useful alternative forms of the power-reducing formulas be replacing "X" with (X/2)

Sin (X/2) = +/- * (1 - cosX) / (2)

Cos(X/2) = +/- * (1 + cosX) / (2)

Tan(X/2) = [ (1-cosX) / (sinX) ] = [ (sinX) / (1 + cosX) ]

* = square rooted

-Graphical Representation of a Half-Angle on the

Unit Circle

-I apologize for the lack of "actual" mathematical signs. I received trouble being able to enable the math widget on my computer. I did the best with the recourses at hand.

5.4 Sum and Difference Trig Formulas


Here are the formulas we have learned:

sin(x+y) = sinx cosy + cosx siny

sin(x-y) = sinx cosy - cosx siny

cos(x+y) = cosx cosy - sinx siny

cos(x-y) = cox cosy + sinx siny

How to derive these formulas:

Now that we know sin(x+y) = cosx siny + sinx cosy

And we know that tanx=sinx/cosx
We can derive tan (x+y)=





















_____________________________________________________________

example problem:

5.3 Solving Trigonometric Equations

To solve a Trigonometric equation use standard algebraic techniques such as collecting like terms and factoring.

The goal is to isolate the trigonometric function involved in the equation.

Sometimes this involves changing sines and cosines in order to isolate one of the variables in the equation.

This is the answer in degree form it can easily be changed into radians if you know your unit circle.

Example 1: Solving a Trigonometric Equation

( In reverse order, look at the part above)

Now that you have used the quadratic formula to find the solutions to the problem you must plug them into your calculator as they are not on the unit circle. In this case you would plug the solution to the inverse of pi to find the answer.

The domain must be restricted on from [0,2pi).

Many other problems need factoring in order to solve them.

Solving Equations

Today we talked about solving equations using algerbra and a small amount of trig.

Because this is a pre-calc class I hope we all know how to solve equations using algerbra, ya know.

Factoring quadratics

What ever you do to one side do to the other

When you take a square root dont forget the + or -

Factoring the sum and difference of Cubes

Just Simple Algerbra Stuff.

There are some exceptions though.

The D: is almost always [0,2pi).

Dont Forget you can substitue using Trig Identities.

Just like you CANT divide by a square root you CANT divide by a trig function. Just take the inverse

You still shouldnt divide by a varrible. factor it instead.

Some easy hints.

Change all of the Trig functions to the same thing such as (sin) or (cos)

treat the Function as the varrible I.E. c=constant Tan(x) or tan(x-c)=just "X"

UNTILL youve finished isolating the varrible then take the inverse.

All of the identities are on the post before mine if you need them.

Every problem starts COmplex and can be reduced down to something simple. dont give up

http://www.youtube.com/watch?v=QS-0C8ink7M

Identity Stuff

RECIPROCAL IDENTITIES

QUOTIENT IDENTITIES

PYTHAGOREAN IDENTITIES

COFUNCTION IDENTITIES

EVEN/ODD IDENTITIES